MAT2705 23S homework and daily class log

Jump to current date! [where @ is located]

Daily lectures and your homework will appear here each day as it is assigned, including some PDF and/or Maple problem solution files or PDF notes, with occasional links to some MAPLE worksheets when helpful to illustrate some points where technology can be useful. [There are 56 class days in the semester, 4 each normal week, numbered consecutively below and labeled by the (first initial of the) day of the week.]

It is important that you read the section in the book from which homework problems have been selected before attempting them.

It is your responsibility to check homework here since most but not all homework exercises are online in the MyLab Math portal entered through BlackBoard. Homework hints and some textbook exercise solutions are also found here. You are responsible for requesting any handouts or returned tests or quizzes from classes you missed. Homework is understood to be done by the next class meeting (unless that class is a test, in which case the homework is due the following class meeting), but the online deadline will be stated as midnight of the due day so that you have time in class or after to ask questions that you did not submit via the Ask Your Instructor online tool. You may correct submitted homework after the deadline without having to request an extension.
[[Occasional homework problems surrounded by double square brackets [[...]] are not in the online homework system but are important to do.]]

Read: HOMEWORK ADVICE;
quiz BlackBoard access/submission

T: (January 17)
DURING CLASS:
Lecture Notes 1.1a: Differential Equations: how to state them and "check" a solution;
summary handout: odecheck.pdf

We will enter the e-text MyLab Math portal and homework software inside the Content page of BlackBoard, as explained in the welcome email message!

New to Maple 3 minute video

AFTER CLASS (THIS IS THE HOMEWORK):
1) Log on to My Nova, choose the Class Schedule with Photos, view fellow students.
2) Go to BlackBoard and look at the class portal and Grade book for our course: you will find all your Quiz, Test and Homework grades here during the semester once there is something to post. Everything we do except for quizzes, tests and grades will take place through our course website although you will access our e-text portal inside BlackBoard to avoid an extra login.
3) Make sure you have Maple 2022 on your local computer, available by clicking here. if you haven't already done so and install it on your laptop when you get a chance (it takes about 15 minutes or less total), If you have any trouble, email me with an explanation of the errors. You are expected to be able to use Maple on your laptop when needed. We will develop the experience as we go.
If you have any trouble, email me with an explanation of the errors.
You are expected to be able to use Maple on your laptop when needed. We will develop the experience as we go.
No previous experience is assumed.
4) Enter the e-text MyLab Math portal if you have not already done so, as explained in the welcome email message!
5) Homework Problems: 1.1: 3, 5, 13, 33
(only a few problems so you can check out our class website and read about the course rules, advice, bob FAQ, etc, respond with your email).
6) By the end of the week, reply to my welcome e-mail from your OFFICIAL Villanova e-mail account (which identifies you with your full name), telling about your last math courses, your comfort level with graphing calculators and computers and math itself, how much experience you have with Maple if any (and Mathcad if appropriate) so far, why you chose your major, etc, anything you want to let me know about yourself that will give me more of an idea about you as a person. [For example, I like to do humorous sketching. and cooking.] Tell me what your previous math course was named (if at VU: Mat1500 = Calc 1, Mat1505 = Calc 2, Mat2500 = Calc3).
[In ALL email to me, try to include the string "mat2705" somewhere in the subject heading if you want me to read it quickly. I filter my email.]
W: Lecture Notes 1.1b: Differential Equations: initial conditions
[extra: initial data: what's the deal?]; [gravity fall example]
1:1: 8, 23 [see Maple plot (execute worksheets by clicking on the !!! icon on the toolbar when necessary)];
formulating DEs: 27 [as in 29 below: make a generic diagram like this one to calculate the slope of the tangent line and set it equal to the derivative .mw; namely the change in y over the change in x between these two points (x,y) and (y,-x) equals dy/dx],
31, 35, 43; perhaps in class together:
[[29. Hint: recall perp lines have slopes which are negative reciprocals, the normal line is perpendicular to the tangent line and passes through the same point on the curve; make a diagram of the given point (0,1), a "generic" function graph curve whose normal at a point (x,y) on the curve passes through (0,1), and draw in the connecting normal line segment between these two points and the perpendicular tangent line, then compute the slope of the normal line from the two points, and then from the negative reciprocal of the derivative value, finally equate the two to get the DE: mw ]].

New Maple users? I would be glad to show you one on one in my office how to get started.

[memorize!: "A is proportional to B" means "A = k B" where k is some constant, independent of A and B]
Proportionality statements must be converted to equalities with a constant of proportionality introduced:
y is proportional to x: y ∝ x means y = k x . [y is a multiple of x]
y is inversely proportional to x: y ∝ 1/x means y = k/x .
y is inversely proportional to the square of x: y ∝ 1/x² means y = k/x²
F: Quiz 1 on checking solutions to DEs, paper copy handed out in class (read Test/Quiz rules): due in class Monday;
Lecture Notes 1.2: First order DEs independent of the unknown
[finding particular antiderivatives interpreted as solving a first order initial value problem illustrated by textbook example problems:
lunar landing example: mw, optional: Swimmer problem example: mw]
1.2 (antiderivatives as DEs): 1, 5, 15,
21 [Hint: write piecewise linear function from graph: v₁(t) for first expression, v₂(t) for second expression, solve first IVP for x₁(0) = 0, second IVP for x₂(4) = x₁(4); example: pdf, mw],
25 (like lunar landing problem example above), 35,
together in class:
41 (first solve bomb drop IVP, evaluate time when it arrives at y = h (target height), then solve projectile IVP with unknown inital velocity, then impose that it reach height h at the given time: game plan)
[[important lesson on choice of units not in MyLab: 43, convert final answer to appropriate units!]].

Send me the email about yourself with your schedule attached please if you have not already done so.

WEEK 2[-1]:
M: Lecture Notes 1.3: First order DEs; Direction (= slope) fields and complications
[directionfields.mw] [ode1-complications]
1.3: [use directionfields worksheet to plot:] 3, 5,
11, 15;
[[in class plus HW drawing exercise: 1.3: 3: hand draw in all the curves with a pencil on the slope field printout.
T: Lecture Notes 1.4a: Separable first order DEs
[example 1: only implicit soln]
1.4 (separable DEs): 1, 4, 9 [use Maple for antiderivative! add absolute value signs for ln integrals],
25, 27 [first expand exponential into 2 factors e^(A+B)=e^A*e^B], 29.
W: Lecture Notes 1.4b: Separable first order DEs
handout on exponential behavior/ characteristic time [cooking roast in oven remarks]
[how to choose exponential plot window];
1.4: 33, 35,
41 [hint: first we need to evaluate the fraction of the total U + L which is only U, namely U/(U+L)],
45 [attentuation of signal--characteristic length],
49 [cooling problem].
F: Quiz 2 (separable DEs and directionfields); read answer key to Quiz 1 (will be returned Monday);
slope field work sheets returned with feedback;
together in class:
1.4: 69 [hanging cable problem; hyperbolic functions are important in DEs! [wiki], so is working with problems involving parameters];
"fun" with Newton's law of cooling not online:
[65 CSI problem (same as the in lecture example and problem 49 but at end we want to find a previous time instead of a subsequent time)].

Catch up with HW over weekend.

WEEK 3[-1]:
M: Lecture Notes 1.5a: Linear first order DEs
online handout: recipe for first order linear DE
1.5: 2, 5, 8, 21, 24 [u-sub integral];
(these involve using rules of ln's and exp's).

For future reference to get Maple soln of a DE (Maple template):
enter DE alone or DE plus initial conditions separated by commas, from context menu at right choose Solve DE, for y(x)
> y ' = x y , y(0)=1
Use function notation to change independent variable:
> y '(t) = t y(t) , y(0) = 1
If you use the d/dx y(x) from the palette, you must use function notation y(x) throughout the DE.
T: Lecture Notes 1.5b: Linear first order DEs:complications
[accumulation functions]
1.5: 15, 24 (more practice);
27 (switching independent and dependent variables),
30 ( just do the linear procedure choosing the antiderivative as the definite integral from 1 to x without evaluating it, choose integrating factor for x > 0 application to IVP).
W: classroom move to Bartley 024 only for today [basement level, first classroom behind common area];
Lecture Notes 1.5c: Linear first order DEs: Mixing Problems [1.5.37 worked by hand]
[these mixing tank problems are an example of developing and solving a differential equation that models a physical situation, and one where we have some intuition];
Solute (salt) plus solvent (water) makes a solution (salt water!); concentration of solute in solution = ratio of solute to solution;
different concentrations equalize when mixed;
1.5: Use Eq. 18 in the book and plug in values of parameters:

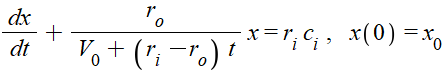
solve by hand, then if necessary check with Maple template;
respectively constant, decreasing, increasing volume cases: 33, 36, 37 (Maple worked solutions with textbook exercise numbers).

Note: we are skipping 1.6: exact DE etc. These are less important, and Maple can solve these when needed anyway. A similar integrating factor technique works for exact equations but where the independent and dependent variables are on an equal footing.
F: Quiz 3 on linear DEs;
Chapter review problems are not in MyLab Math;
1.R (review problems are not online!): in class we classify some of the odd problems 1-35 but don't solve them;
[[ solve 25 [it can be done in two ways by expanding out the square on the RHS before integrating with integration constant C or by using a u-substitution with integration constant K; express K in terms of C by combining the two terms in the K solution [use the identity for expanding out (x+1)³ = x³+3 x² +x+1] and then comparing with the C solution];
both separable and linear:
solve 31 in two ways and compare the results;
solve 35 in two ways and compare the results;
in both cases ---different constants enter your expressions, show how they agree]].

Catch up on improving HW score. Read answer key to Quiz 2.

WEEK 4[-1]: Chapter 2 is just applications of chapter 1.
M: Lecture Notes 2.1a: First order DEs: Logistic Equation
handout on solution of logistic DEQ [Maple: directionfield, integral formula, shape];
2.1 (only the logistic DE part of the reading is necessary):
5 (resolve the DE this one time following the steps in the Lecture Notes/handout or just use the final formula),
17 (see Maple worksheet for setup problem 15);
21 (initial value of population and its derivative given instead of population at two times, need to set k and P(0) with two conditions),
23 (Rewrite in form dx/dt = k M x - k x² = k x (M - x) to identify first k and then M to then use the logistic solution formula.).
T: Lecture Notes 2.1b: First order DEs: More models, Separable: f(y)
2.1 (other population models):
handout on DE's that don't involve the ind var explicitly; cubic example;
11 ["inversely propto sqrt": use β = k₁/P^(1/2), δ = k₂/P^(1/2) in eq.(1),
beta and delta are fractional (logarithmic derivative) birth/death rates;
this leads to P' ~ P^(1/2)]
13 [P' ~ P^2],
39 [oscillating growth, plot pure exponential and solution together, see Maple worksheet];
optional: growth rate cutoff: 30,31 (not online).
W: Lecture Notes 2.3: First order DEs: acceleration models
2.3 (acceleration-velocity models: air resistance, section resistance proportional to velocity only):
1, 3,
9 [remember weight is mg, so mg = 32000 lb determines mass m = 1000 in USA "slug" units, convert final speed to mph for interpretation!],
19.
F: Quiz 4 on logistic equation; Read answer key to Quiz 3 (characteristic time only applies to single exponentials);
Lecture Notes 2.4: First order DEs: numerical DE solving with Euler's method
[Euler Tutor template for HW]
2.4: 1, 7
[[complications: 27]]

WEEK 5[-1] Test 1 this week: Wednesday
M: Lecture Notes 3.1: linear systems of equations: elimination, DEs;
[inconsistent 3x3 system; DEs lead to linear systems];
Read 3.1 on linear systems (review of high school topic solving 2 or 3 linear equations in 2 or 3 variables),
3.1: 2x2: 3, 5, 7; 3x3: 9; 2x2 IVP: 23, 27.
T: 3.2: Lecture Notes 3.2: matrices and row reduction "elimination";
handout on RREF (Reduced Row Echelon Form, section 3.3) [see bob's examples 2, 3 using Maple];
3.2: 1, 5, 7, 9 [these are partially reduced, only requiring successive backsubstitution to solve, you can do this on paper];
11,13,15,
[you may do these by hand on paper or use step-by-step row ops with the MAPLE Tutor; we ALWAYS want a complete reduction, you can insert the complete Gauss-Jordan reduced row echelon form (RREF) to get practice] ;
you must learn a technology method since this is insane to do by hand after the first few simple examples;
23 [matrix with a parameter, reduction depends on value];
in class we reduce two matrices in this Maple file using the LinearSolveTutor.

Happy Valentines Day! hearts with Maple
W: Test 1. Arrive promptly to get started quickly. One separable DE, one linear DE.
Read Test Instructions; Remember bob is trusting you to observe our Villanova honor system.
F: 3.3: we catch up with RREF details, no partial reductions as in 3.2!;
Lecture Notes 3.3: Row reduction solution of linear systems;
online handout on solving linear systems example [.mw];
we always want to do a full [Gauss-Jordan] reduction, not the partial Gauss elimination reduction also described by the textbook;
in class practice worksheet;
3.3:
reduce: 3, 9, 11, 14, 17, 19,
solve: 21, 23, 29 [as in practice worksheet]
You can use this template to invoke the Gauss-Jordan EliminationTutor for the homework problems.

WEEK 6:[-1]:
M: Lecture Notes 3.3: Balancing Chemical Reaction Application
(application of integer matrices in homogeneous linear systems) [solutions]
Quiz 5Not! take home, due tomorrow.
T: Lecture Notes 3.4: Matrix operations
3.4: 1, 5, 9, 2, 11, 13, 15, 17, 19.
[online matrix multiplication: handout, Maple]
W: Lecture Notes 3.5: Matrix inverses; [2x2 example]
3.5: 1, 5, 7, 9 (use the 2x2 inverse formula to do by hand for practice);
11, 19 (row reduce augmented matrix, then check with Maple inverse);
23 (multiply both sides by inverse matrix to solve; Maple check).
.
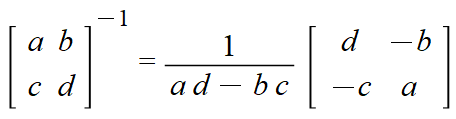Switch diagonal entries. Change sign off-diagonal entries. Divide by determinant.
Always check inverse in Maple if you are not good at remembering this.
F: Quiz 5; Quiz 5NOT back: answer key;
Lecture Notes 3.6: Matrix determinants; [examples]
[forget minors, cofactors, forget Cramer's rule (except remember what it is when old-fashioned texts refer to it), use Maple to evaluate determinants; we only need row reduction evaluation to understand determinants];
3.6: use a few SwapRow, AddRow ops with the G-J reduction Tutor to find upper triangular form for a few, or just use Maple (Standard Operations) to evaluate the determinant:
7 (easy! AddRow leads to zero row, zero value),
9, 10, 13,
29 (Cramer's rule just to be aware);
catch up on old HW.

WEEK 7[-1]
M: Lecture Notes 4.1: R^2 and R^3: Linear independence;
R^n spaces and linear independence of a set of vectors [vector space rules];
now we look at linear system coefficient matrices A as collections of columns A = < C₁| ... |C_n >,
matrix multiplication on the right by a column of coefficients as taking linear combinations of those columns,
A x = x₁ C₁ + ... + x_n C_n
and solving homogeneous linear systems of equations A x = 0 as looking for linear relationships among those columns (nonzero solns=linear rels) ;
4.1: 5, 7; 13; 15, 17; 19, 23; 29, 31, 33 [example].
T: Lecture Notes 4.2: Vector spaces and subspaces [in class exercise: solution];
(homogenous linear systems define subspaces!);
[note only a set of vectors x resulting from the solution of a linear homogeneous condition A x = 0 can be a subspace of a vector space; interpretation: lines, planes, ..., hyperplanes through the origin];
4.2: 1, 3, 7, 11; 15, 21.
W: 4.3: Lecture Notes 4.3: Span of a set of vectors > extended exercise from yesterday;
Read answer key to Quiz 5;
so far: linear independence (A x = 0), or express a vector as a linear combination of other vectors (A x = b) ;
now give the name span to the subspace of all linear combinations of a set of vectors, like we get from solving A x = 0;
to solve A x = b is express b as a linear combination of the columns of A if b lies in the span of those columns.
4.3: 1, 3, 5, 7; 9,, 13; 17, 21.
F: Lecture Notes 4.4: Bases; [handout: new coordinate grids] [pick your own basis] [subspaces?]
online note on linear combinations, forwards and backwards [maple to visualize]
4.4: 1, 3, 5 [zero row!], 7;
9 [this is in already reduced form, find solution as in class example, pull apart to find coefficient vectors of parameters, repeat for next 4 problems reducing first in the last 3],
13 [equations already in reduced form! express all 4 variables in terms of free variables c, d, read off coefficients of those two free variables];
15, 17 [use technology for all row reductions].

Spring Break. enjoy and be safe.

WEEK 8[-1]:
M: new coordinates on the plane workshop. Complete for HW if not finished in class.
Read why we are doing this here.
T: 4.7 Lecture Notes 4.7: Vector spaces of functions;
bridge to vector spaces whose elements are functions.

Part 2 of course begins: transition back to DEs:
read the 4.7 subsection on "Function Spaces" and then examples 3, 4, 6, 7, 8;
[if you are curious, example 5 illustrates the partial fraction decomposition needed in engineering integration but Maple does this easily];
Optional worksheet explaining the vector space of (at most) quadratic functions [quadratics.mw];
4.7: 9, 11 [is the condition on the coefficients a linear homogeneous condition? only if so do these form a subspace]
14 [note that any two (nonzero!) functions of x that are not proportional with a constant ratio are automatically linearly independent],
15 [Hint: solve c₁(1 + x) + c₂(1 - x) + c₃(1 - x²) = 0, for unknowns c₁,c₂,c₃; are there nonzero solutions? if not, these are linearly independent polynomials, in terms of which any quadratic expression in x can be written],
18 [are the two functions proportional? if not, they are linearly independent].

Monday exercise on grids completed here.
W: Lecture Notes 5.1a: 2nd order linear DEs: an intro;
read 5.1 up to the subsection on Linear Independent Solutions (this is a long section);
Memorize: y ' = k y < -- > y = C e^{k x} (from chapter 1)
y '' + ω² y = 0 < -- > y = C₁cos(ω x) + C₂ sin(ω x)
[when x is a time variable, "omega" = ω is the angular frequency "radians per time unit" ]
5.1: 1, 3, 9, 11 [verify solutions, then impose initial conditions];
17 [nonlinear DE does not allow scaling of solutions]
F: Quiz 6;
5.1: W: Lecture Notes 5.1b: constant coefficient 2nd order linear homogeneous DEs;
repeated root plot [problem 49 worked here is useful for working with exponentials];
5.1[after Linear Independent Solutions subsection]: 33, 35, 38, 39.

WEEK 9[-1]:
M: Lecture Notes 5.2: The Wronskian and higher order constant coefficient 2nd order linear DEs;
[Maple Wronskian][example 1] [example 2]
5.2: 1, 7, 13, 17, 23.
T: Lecture Notes 5.3.0: Complex arithmetic and complex exponentials
handout on complex arithmetic, exponentials
[Maple commands; the complex number i is uppercase I in Maple];
no homework.
W: Test 2 on chapters 3, 4. no 2d grid plot this time.
F: Lecture Notes 5.3a: higher order constant coefficient linear homogeneous DEs and complex exponentials: distinct roots;
(use Maple to find roots of characteristic equation).
5.3: 1, 9, 17, 23, 33.

WEEK 10[-1]:
M: Lecture Notes 5.3b: higher order constant coefficient linear homogeneous DEs: repeated roots;
5.3: 5, 13, 25, 35 [ignore instructions to use one solution to find more; just find roots with Maple!]
T: Lecture Notes 5.3c: sinusoidal and decaying sinusoidal functions;
To plot envelope functions of decaying amplitude together with the decaying oscillation, do:
> plot( [A e^{-k x}, -A e^{-k x}, e^{-k x} ( c₁cos(ω x) + c₂ sin(ω x) )], x=0..10)
where <c₁,c₂> = <A cos(δ), A sin(δ)>
transforms Cartesian to polar coordinates in the parameter plane (draw a diagram).
For HW try this with y = e^-x/2 (-3cos(10 x) +5 sin(10 x) ) .

Test 2 back in class; see answer key.
W: Lecture Notes 5.4: Linear homogeneous 2nd order DEQ with constant positive coefficients (damped harmonic oscillators);
5.4: read worksheet for help on this problem:
3: Undamped oscillator: y '' + ω² y = 0 < -- > y = C₁cos(ω x) + C₂ sin(ω x) [omega is a small integer for this problem]
frequency vocabulary: when x is a time variable, "omega" = ω is the angular frequency "radians per time unit" as opposed to just "frequency" f = ω/(2π ) or "revolutions or cycles per time unit" as in physics, but in our class we will just say "frequency" for ω, assumed to be expressed in radians per time unit or converted to revolutions per time unit as convenient;
see also damped harmonic oscillators and RLC circuits, and Hertz (computers now have GHz clock speeds), "cycles per second"];
13 (goal: maximum positive displacement setting derivative to zero);
solve these next problems by hand, determine phase-shifted form, then plot undamped and damped solutions together:
15 overdamped,
17 critically damped,
19 underdamped.
Here are the textbook parameter exercises solved so you can edit the plots to help pick out the online plot choice after solving these problems by hand. The evaluation of the numerical phase shifts is also explained for each problem.
F: Quiz 7 on underdamped harmonic oscillator;
In class help for 5.4 HW.

WEEK 11[-1]:
M: Lecture Notes 5.5: NON-homogeneous 2nd order DEQ with constant coefficients
summary handout on driven (nonhomogeneous) constant coeff linear DEs;
[final exercise on this handout sheet: pdf solution];
5.5: 1, 3, 8 [just express cosh or sinh in terms of exponentials], 10, 33, 35;
[not many of the book RHS driving functions are physically interesting here;
we will not cover "variation of parameters"; the book presentation of the method of undetermined coefficients is a recipe with little justification (educated guess), instead the undetermined coefficient method handout shows exactly how and why one gets the particular solutions up to these coefficients].
T: Lecture Notes 5.6a: Driven damped harmonic oscillators (over 2 days);
[today example with resonance calculation side by side; .mw],
5.6: 11 [ HW portal wants phase-shifted cosine form for both steady state and transient sinusoidal factor, phase shift between 0 and 2 Pi, decimal solutions, then plot].
W: Lecture Notes 5.6b: Driven damped harmonic oscillators: special cases;
summary handout on damped harmonic oscillator driven by sinusoidal driving function [1 sheet printable version];
resonance plots; [only for fun: beating!]
5.6: 1, 3 [ undamped solutions driving frequency not equal to natural frequency],
17 [resonance calculation with reasonable numbers].

Please catch up on HW over break.

Optional.
Watch the 4 minute Google linked video of resonance NOT! (but see the engineering explanation linked PDF for the detailed explanation):
Tacoma Narrows Bridge collapse (resonance NOT!): Wikipedia; Google (You-Tube video)] (4 minutes);
a real resonance bridge problem occurred more recently: the Millenium Bridge resonance (5 minutes).

Easter Recess:

see Quiz 7 answer key

WEEK 11[+1]:
T: chapter 5 review, homework catchup;
Quiz 7 extended [answer key] to show examples for online homework exercises for the chapter for when you are reviewing for Test 3 [nonhomogenous case, resonance, beating, phase-shifted cosine form].
W: Transition back to linear algebra:
Lecture Notes 6.0 Eigenvectors and eigenvalues of 2x2 matrices;
Lecture Notes 6.1a: Eigenvectors and eigenvalues (last page tomorrow 3x3 example);
bob uses the Maple worksheet EigenExploreDrag2.mw to graphically determine the eigenvectors and eigenvalues of some 2x2 matrices.
[in the lecture bob first explains why we want to do this with a motivating example of a coupled system of DEQs and its directionfield]
6.1: 1, 2, 7.

Maple context menu: Eigenvalues,etc, Eigenvectors (gives both eigenvalues and eigenvectors).
F: quiz 8 on eigenvectors;
Lecture Notes 6.1b: Eigenvectors and eigenvalues: n>2 and more (linear independence, complex eigenvectors: examples);
6.1: 15, 19, 23 (upper triangular so diagonal values are eigenvalues!),
31 (complex eigenvalues)
[do everything by hand for 2x2 matrices;
For 3x3 or higher, go thru process: use Maple determinant: |A-λI| = 0 (right click on matrix, Standard Operations), then solve to find characteristic equation and its solutions, the eigenvalues, back sub them into the matrix equations (A-λI) x = 0 and solve by rref, backsub, read off eigenvector basis of soln space, NO POLYNOMIAL DIVISION! compare to Maple's Eigenvector result, from the context sensitive menu].
Ignore polynomial division discussion (as useless as long division!), use technology for roots of polynomials and determinants!

WEEK 12[+1]: takehome test 3 during week 13
M: Lecture Notes 6.2: Diagonalization [short version in class];
summary: diagonalization; [diagonalize: 3x3 examples];
6.2: 3, 10, 13, 19, 25 [use Maple for det and solve for eigenvalues, then by hand find eigenvectors, use Maple for matrix multiplication; check with Eigenvalues etc; online eigenvector matrices are all integer valued, so perhaps it is worth scaling up your eigenvectors to integer values].
in class this handout exercise:
For the matrix: A = <<1|4>,<2|3>> entered by rows, use Maple to find the eigenvalues and the basis changing matrix B = <b1|b2> of corresponding eigenvectors, evaluate the matrix product A_B = B^-1A B to see that it is diagonal and has the corresponding eigenvalues in order along the diagonal, and use the coordinate transformations x = B y and y = B^-1 x to find the new coordinates of the point x = <x₁, x_2> = <-2,4> in the plane. Then make a grid diagram with the new (labeled) coordinate axes associated with this eigenbasis (labeled also by the eigenvalue λ = <value> ) together with basis vectors and the projection parallelogram of this point.
T: Lecture Notes 7.3a: 1st order linear homogeneous DE systems: real eigenvalues:
(notes are a summary of what we already done with a concrete 2x2 example);
just use Maple to get eigenvalues and eigenvectors, form linear combination of eigenvalue exponentials and eigenvectors to get general soln
7.3:2x2 with direction field
5 (but no need to plot, use eigenline directions and signs of eigenvalues for arrow directions along eigenlines as explained in Maple worksheet);
3x3: 17.
W: 7.3 Lecture Notes 7.3b: 1st order linear homogeneous DE systems: complex eigenvalues: [short version in class]
Not on test 2!
7.3: 15 [no need to plot, check directionfield arrow A x direction along positive vertical axis say at x1=0, x2 = 1], 25.
F: Take home test 3 (on material thru day 47) begun in class; due next Friday in class;
please read the test rules;
two problems from section 5.6: with damping initial value problem, resonance condition (like quiz 7, extended example)
and a real eigenvalue DE system initial value problem.

WEEK 13[+1]:
M: Continue working on Test 3 in class.

Don't forget Quiz 8 Answer Key online.
T: Lecture Notes 7.3d: mixing tanks, etc; [multicompartimental models eqns];
[open flow thru system with real eigenvalues, closed system example 4 flow thru system with complex eigenvalues];
7.3: 33 (open, real, unassigned), 37 (closed, complex USE AS TEMPLATE, you might need "evalc" on your eigenvector matrix to convert to Re plu Im parts);
use eigenvalues and eigenvectors from Maple, write down linear combination of eigenvalue exponentials times eigenvectors as general soln (plus Re,Im aparts with complex case), then impose initial conditions. QUICK. no need to plot, just pick out the plot with the correct asymptotes for the 3 variables.
W: 7.5a: last topic: undamped coupled harmonic oscillator systems: undriven case:
Lecture Notes 7.5a: mass spring systems; [figure8curve.mw];
7.5: 3, 5 [Maple quick check template: epc4-7-5-template.mw]
you just have to identify the natural frequencies ω_i = sqrt(-λ_i ) of the accordian (opposite sign component eigenvectors) and tandem (opposite sign component eigenvectors) modes.
F: Test 3 due in class unless you request an extension over the weekend by email;
7.5b: undamped coupled harmonic oscillator systems: driven case;
Lecture Notes 7.5b: driven mass spring systems (pages 3-4 today); [again figure8curve.mw];
result for the system in the previous class exercise driven by a specific frequency oscillating force :
2mass2spring-short-driven.mw;
(this is the last final exam topic, stopping short of the resonance calculation with general frequency):
use the template epc4-7-5-template.mw to evaluate the matrix and eigenstuff,
7.5: 9;
only eigenvalues and eigenvectors necessary to identify eigenfrequencies and amplitude ratios/signs, but the particular solution via the method of undetermined coefficients is needed to identify the response vector amplitute ratios and relative signs:
11 (initial conditions irrelevant), 13 (solution common for everyone; earthquakes? remove last spring for top of building)

WEEK 14[-1]:
M: 7.5 undamped couple harmonic oscillator systems: resonance;
Lecture Notes 7.5b: driven mass spring systems (page 5 today).
no more homework, catch up: everyone still needs to do 7.5b online.
T [Fri schedule]: final exam shortened practice exercise.
@ W [Mon schedule]: CATS evaluation at beginning of class.
Test 3 back;
How to handle damped couple harmonic oscillator systems? (for the curious);
dynamic damping video (for fun, 9.5 minutes, dynamic damping).

CHECK Black Board grades updated this morning.

Homework assignment catch up possible till end of exam period,
notify me if you are still working at it towards the end of exam period.

*MAPLE homework log and instructions [asterisk "*" marked homework problems]

Test 1: week 5
Test 2: week 9
Test 3: Take home out-in week 12

FINAL EXAM: (not cumulative)
2705-04 MWF/T 10:40: Tues, May 9 02:30 pm - 05:00 pm
2705-05 MWF/T 11:45: Thurs, May 11 02:30 pm - 05:00 pm
[switch okay with permission]

MAPLE and G.Calc. CHECKING ALLOWED FOR QUIZZES, EXAMS

5-jan-2023 [course homepage] [log from last time taught]