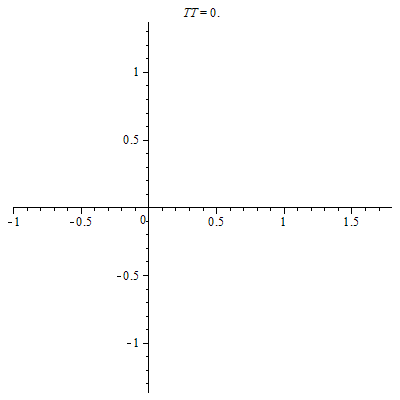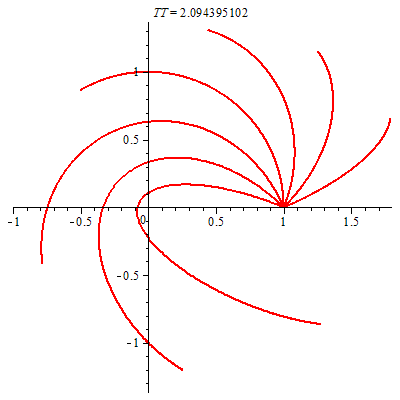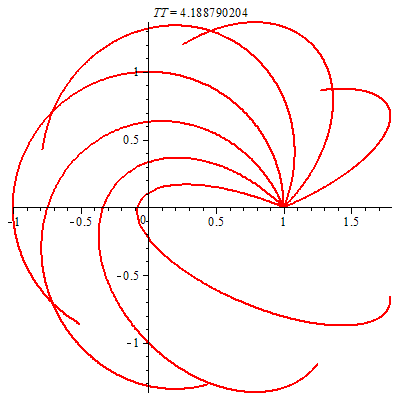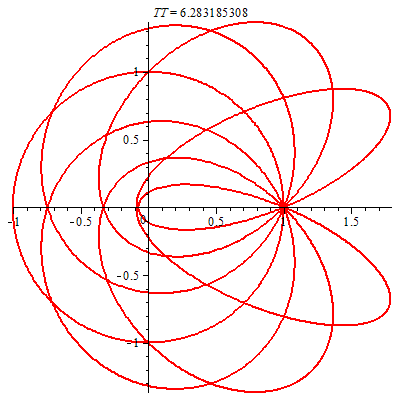
Friday, November 18, 2011 3:30pm Mendel 341
Villanova Physics/Astronomy Debate:
Title: gravity orbits and donut geodesics: the same problem nicely handled by Maple
Speaker: bob Jantzen, dept of math and stat (undercover physicist), villanova university
Abstract: The problem of free motion constrained to
a torus (donut surface) as well as describing planar orbits in nonrelativistic
and relativistic gravitation are really the same mathematical problem. This
system can be understood using elementary ideas of energy and angular momentum
conservation, and Maple can easily display the orbits of either problem for a
given choice of initial conditions.
Disclaimer: Obviously special purpose orbit plotting software can make a nicer
animation, but Maple allows you to study all aspects of the problem---symbolic,
numerical, graphical---on the same platform with pretty good typesetting for the
documentation.
Acknowledgement: Without my friend and colleague
Klaus Volpert
sharing this problem with me initially, it never would have come to my
attention. Thanks, Klaus.
Web pages:
Torus animation page, surfaces of revolution
Maple worksheets: surfaces of revolution,
torus geo game [if you have Maple on your computer,
these will open up from clicking on these links.]
[for really big Maple worksheets with more detail than you want to see: go to
torus geos main page]
The nonrelativistic Kepler problem [WIki1, Wiki2] has the property that the period of an elliptical orbit is independent of the angular momentum and depends only on the energy, so that for a fixed radius starting point, all the orbits with the same initial speed have the same period as the circular orbit. If we pick unit parameters for the radius and the Kepler force coefficient, the unit circular orbit at unit speed takes a time 2 π to make one revolution. Here is an animation of a family of equally spaced initial angle initial data for that unit speed. [Choppy motion to avoid putting really large graphics files on my website.]
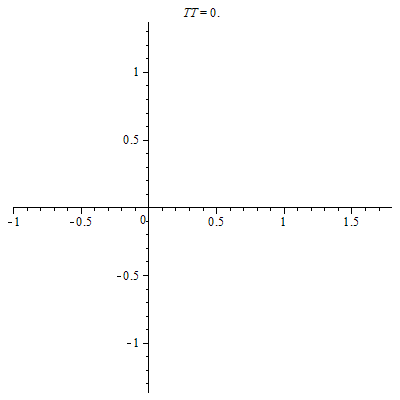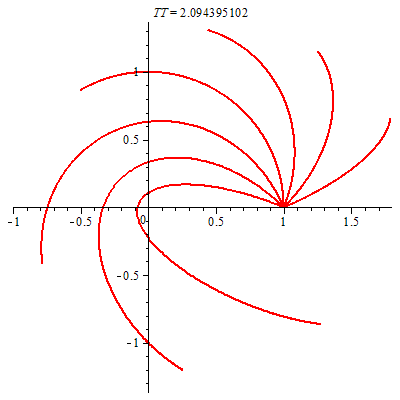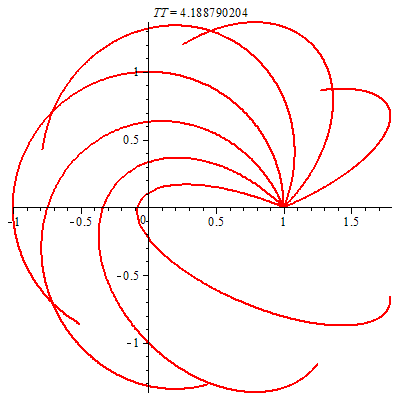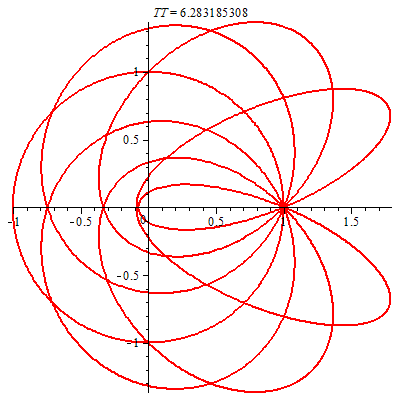 |
 |
| effective potential for radial motion: gravity plus centrifugal potential |
For the relativistic Kepler problem [WIki] one can see the precessing elliptical orbit. Here are four loops precessing in the counterclockwise direction.
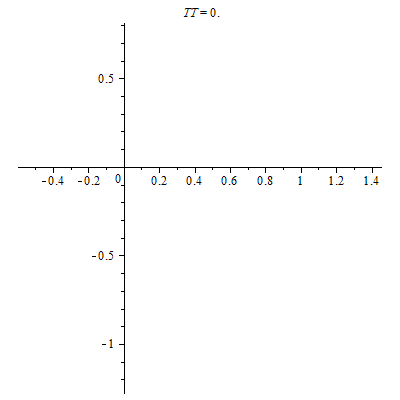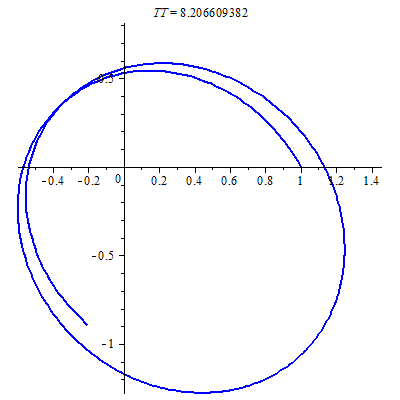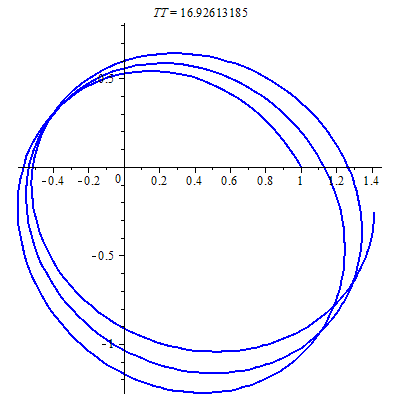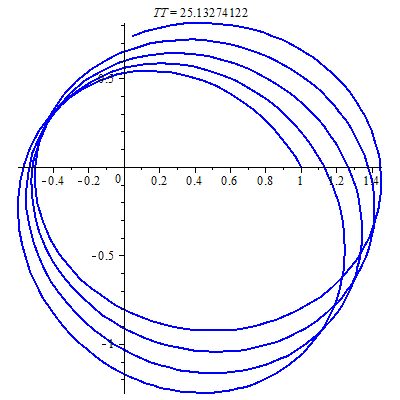
For details see section 8.4 of dr bob's elementary differential geometry.