Abraham, Lorentz, (Poincare), Fermi and Gauss (and a whole lot of others): classical electromagnetic mass, a pedagogical example  advertised as: Abraham, Lorentz, Fermi and Gauss: energy and momentum in classical electrodynamics bob jantzen [not Robert Johnson as advertised in Campus Currents :-) ] Villanova University Physics talk, 3:00pm, Friday, October 26, 2012 Mendel 258 [refreshments at 2:45] Abstract. Gauss's law is pretty important yet we don't teach it here at Villanova in multivariable calculus. We almost get to the Frenet-Serret torsion, but understandably stop short. The latter explains the Thomas precession of the electron. The classical model of the electron developed in the early days of special relativity by Abraham and Lorentz is a nice pedagogical example of the importance of Gauss's law in the 4-dimensional spacetime context. I will try to show how Gauss's law, together with Maxwell's equations,  leads to a conserved energy-momentum 4-vector for the free electromagnetic field (perhaps familiar to professors) and what it does imply for the nonvacuum field of a classical electron (not so familiar, hence Fermi in the title).  I will try to keep undergraduates in mind as I present the details.

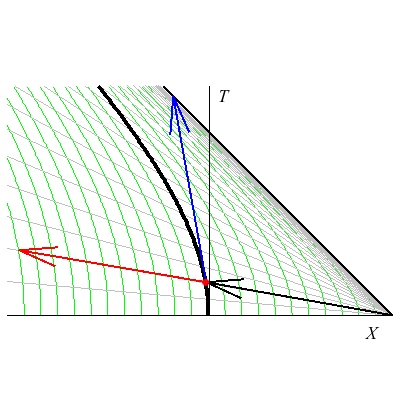electron accelerating backwards due to forward constant electric field (hyperbolic motion)

• alfg-beamer.pdf [LaTeX beamer PDF presentation (zipped file with PDF graphics)]
• hyperbolic.htm [cute video about hyperbolic rotations = Lorentz boosts]
• helix-lorentz.mw [Maple worksheet studying the Lorentz helix, the world tube of a circularly rotating electron in 3d Minkowski spacetime]
• specialrelativity.mw [Maple worksheet generating many of the graphics]

Some of the history behind this:

History of Special Relativity and Lorentz transformations
1905 Lorentz transformations (named by Poincare)
1905 Einstein Special Relativity
1907-1908 Minkowski spacetime diagrams

History of Electromagnetic Mass: classical electron Coulomb field has mass like properties, "4/3 problem"
1881 JJ Thompson
1902 Abraham (sphere period, so not relativistically invariant)
1904-1904 Lorenz (contractile electron: sphere in rest frame)
1905-1906 Poincare stresses (stability)
1911 Max von Laue (closed  systems)
1922 Enrico Fermi (Born rigidity correction of Abraham-Lorentz model)
1960 Rohrlich rediscovery
1983 Schwinger (Poincare stresses)
 

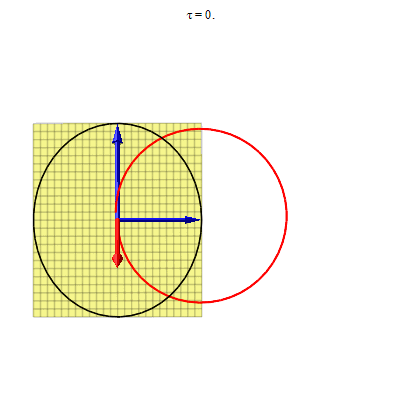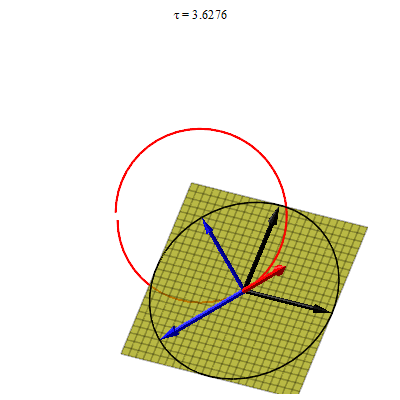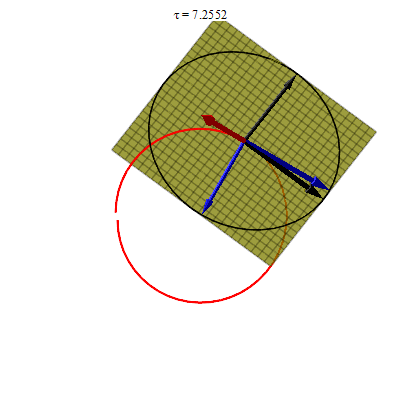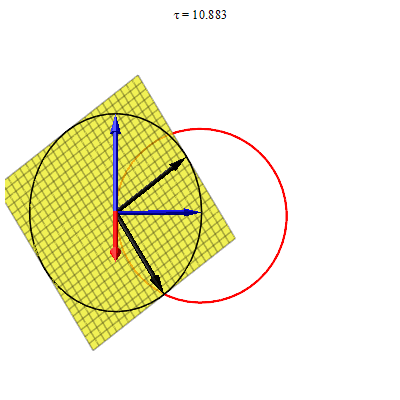
The spacetime diagram of the world tube of a spherical electron shell in circular orbit undergoing Born rigid motion as assumed by Fermi (suppressing one spatial dimension, so showing the equatorial plane circle of the electron sphere) is basically relativistic corkscrew pasta, here shown with the central inertial time axis (blue). The horizontal grid lines on the surface are the local rest spaces (planes) while the vertical grid lines are the world lines points fixed on the circular cross-sections which are fixed in the Fermi spatial coordinate grid.

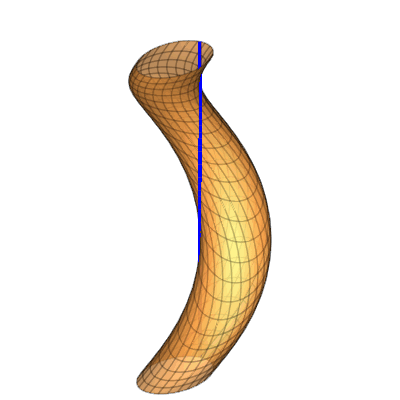
The spatial Fermi coordinate grid in the local rest space (plane) tries to resist the rotation about the central axis due to the twisting of the world tube, but ends up rotating anyway, following Fermi-Walker transport, which corresponds to aligning the grid with torque-free gyroscope directions.

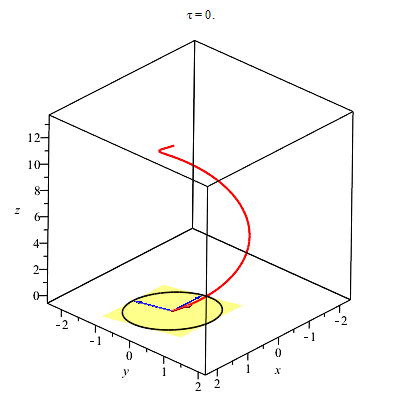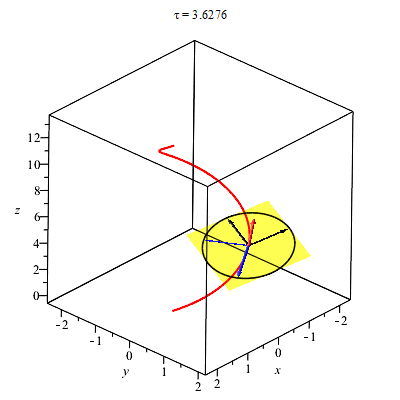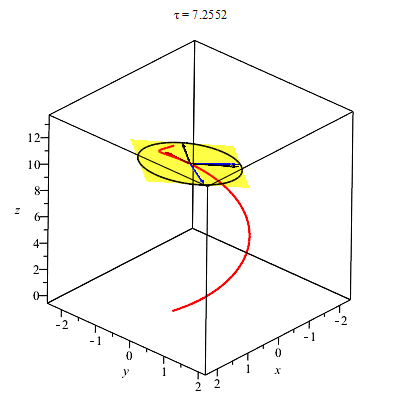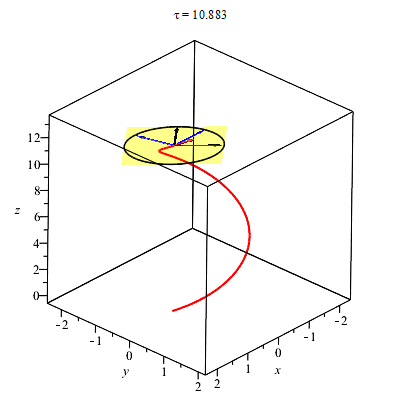
The 4-velocity vector is shown in red (the unit tangent), the 4-acceleration vector points towards the central time axis and is aligned with the unit normal, which is shown in blue together with the binormal  vector in blue, while the Fermi-Walker "locally nonrotating axes" which coincide with them at t = 0 are shown in black. The yellow square representing the local rest space (plane) is aligned with the Fermi-Walker axes.

Since the spin angular velocity of the FW axes moving in the retrograde direction with respect to the acceleration 4-vector is larger than the orbital frequency by a Lorentz gamma factor,  those axes rotate backwards at the difference frequency. This is the Thomas precession of the classical electron in circular orbit around a nucleus. For the speed of half the speed of light, the gamma factor is only about 1.15 and the net angle per revolution of this precession of the FW axes is about 55 degrees. Here is a top view, showing the Lorentz reverse contraction of the yellow square in the direction of motion (due to projection rather than length measurement) and the Thomas precession of the black FW axes.

If you find this interesting maybe you would like to take my 9:30 MWF Differential Geometry class Spring 2013 semester?

Another interpretation of this surface is as an example of a toy model for string theory. The tubular world sheet is a timelike surface in the spacetime containing one timelike direction and one spacelike direction, and is the history of a ``closed string" (the circular loop of the construction) as it moves in spacetime. The horizontal plane cross-sections of the surface are the string as seen by the inertial observer at rest in this coordinate system at a moment of its inertial time. An accessible discussion of such a toy model may be found in a recent book Differential Geometry and Manifold Theory by Stephen T. Lovett.