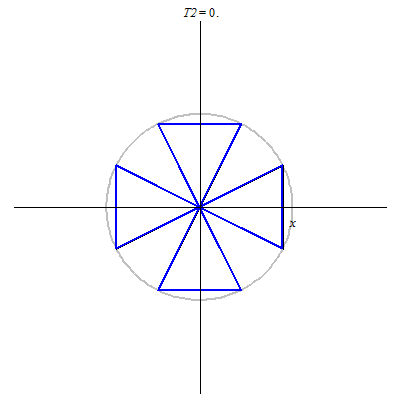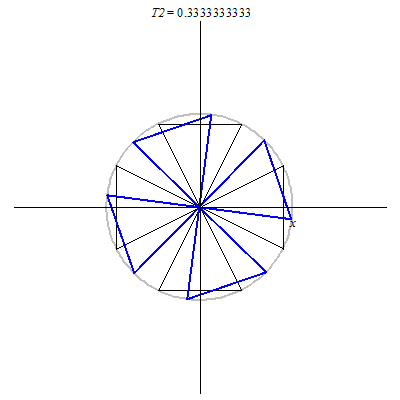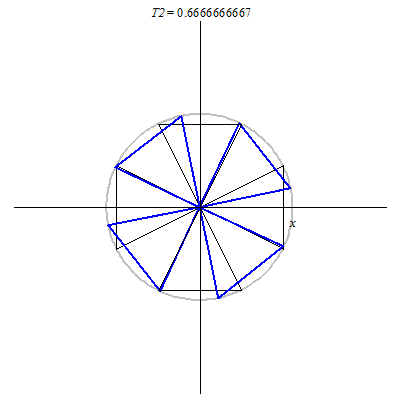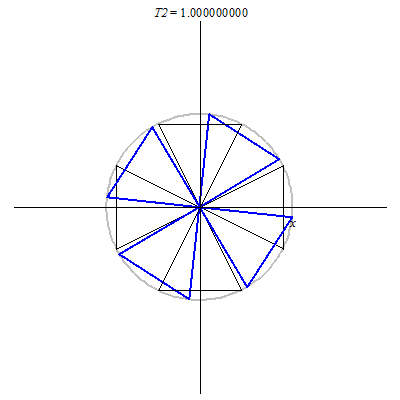
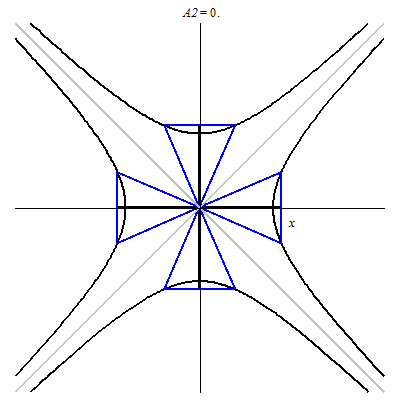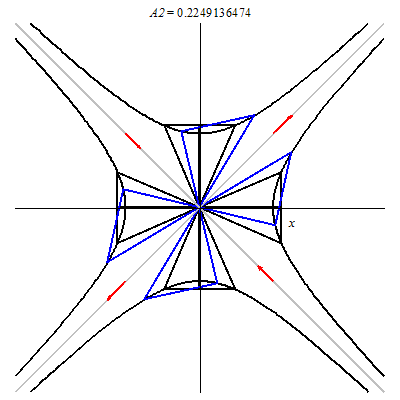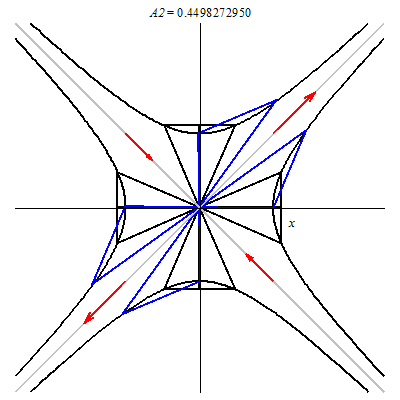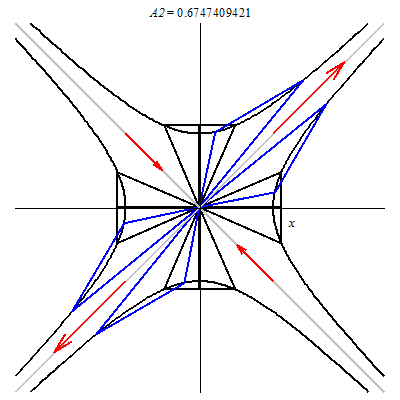
Trigonometry is based on the geometry of the unit circle x2+y2 = 1 in the Euclidean plane. Hyperbolic geometry is based on the geometry of the pair of unit hyperbolas in the Lorentz plane: x2 - y2 = 1 with vertices on the horizonal axis and -x2+y2 = 1 with vertices on the vertical axis.
Here we compare an active counterclockwise rotation of the Euclidean plane by an increasing positive angle with an active hyperbolic rotation (pseudo-rotation = "boost") of the Lorentz plane by an increasing positive velocity parameter. This shows how the points on the blue triangles move under these tranformations. Animated GIFs courtesy of Maple.
 |
 |
| a rotation of the Euclidean plane | a pseudo-rotation of the plane |