solution of a second order coupled system of ordinary
differential equations for the position coordinates on the surface
of a curve which locally minimizes the length of the curve segment between
successive points on it
(called a geodesic)
 |
 |
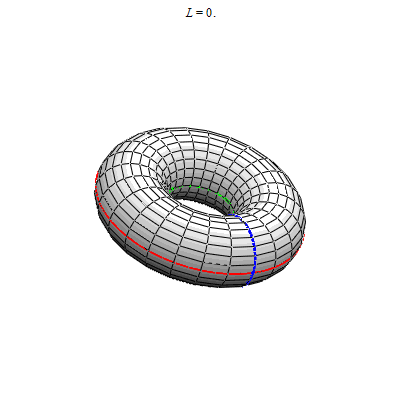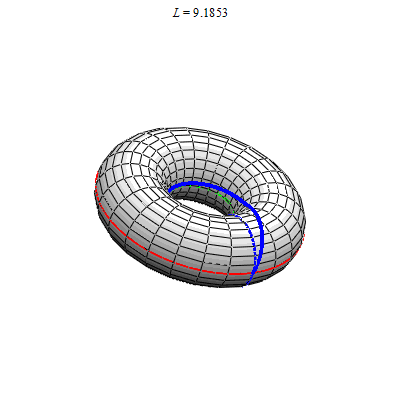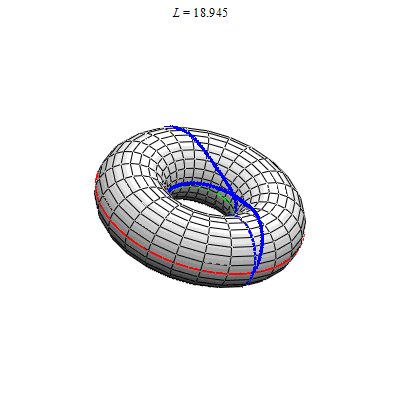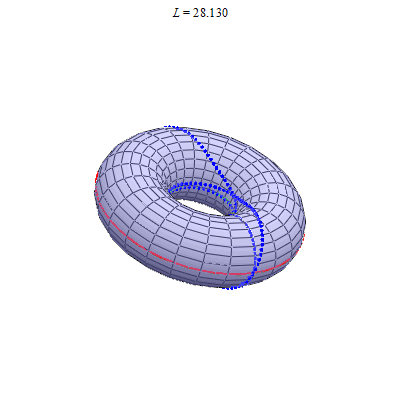
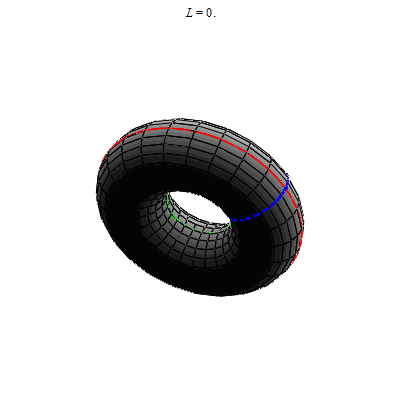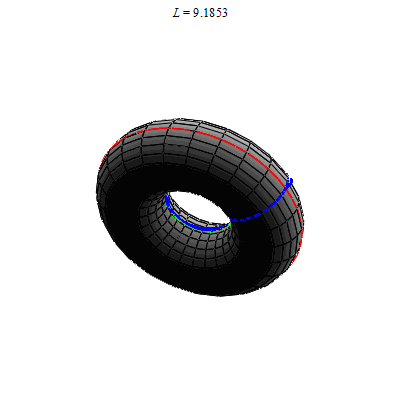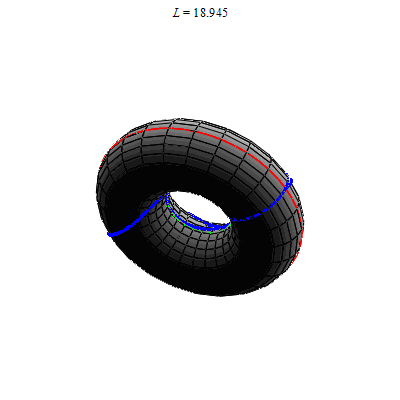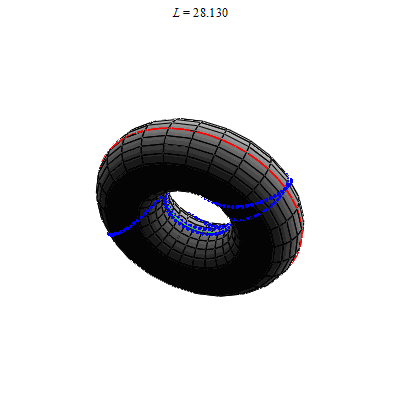
The grid of circles which are plane cross-sections through the vertical axis of symmetry (lines of longitude) and the horizontal circles around the vertical axis (lines of latitude) is directly analogous to the natural grid on the sphere. The vertical blue circle is like the Greenwich line, while there is an outer equator (red circle) and an inner equator (green circle).
The curve starts at the intersection of the blue and red circles aimed upward slightly to the right of the blue circle. It winds around the inner hole of the torus just above the inner equator one loop and then comes back over the top and then slips underneath the bottom side where it repeats the mirror image of the top behavior (one loop around the hole under the inner equator) and finally returns exactly to its starting point (so that it is a closed curve). This is a very special curve, since usually such curves never return to their starting point.
Indeed it makes 1 complete oscillation threading the hole and returning to the outer equator while making 3 revolutions around the vertical symmetry axis through the central hole of the torus, but not passing through the inner equator on the first and last pass (it only "skims" the inner equator!), making it a [1,3;0] class closed geodesic. A closed geodesic can be characterized by the triplet [m,n;p] making n revolutions about the vertical axis, while making m oscillations away from the outer equator, either passing through the inner equator (p = 1) or not (p = 0), letting [0,1;1] describe the exceptional inner equator.
For more details than you want to see, go to the torus page. Or if you want to play the "torus game" see the torus game page.